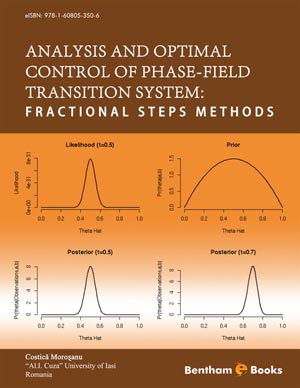
Abstract
Two topics will be covered in this Chapter:
• numerical approximation of the solution of nonlinear phase-field transition system;
• numerical approximation of boundary optimal control, governed by phase-field transition system. For both subjects we consider the phase-field system with non-homogeneous Cauchy-Neumann boundary conditions:....
Keywords: Nonlinear parabolic systems, free boundary problems for PDE, optimal control, inverse problems, methods of approximations based on necessary conditions, numerical analysis, finite difference methods, finite element method, fractional steps method (Lie-Trotter product formula), stability of numerical methods, computer science, analysis of algorithms, methods of gradient type, phase changes, mathematical modeling, applications in engineering and industry.